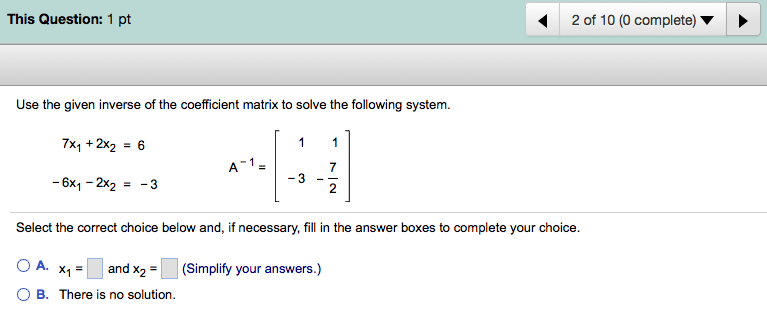
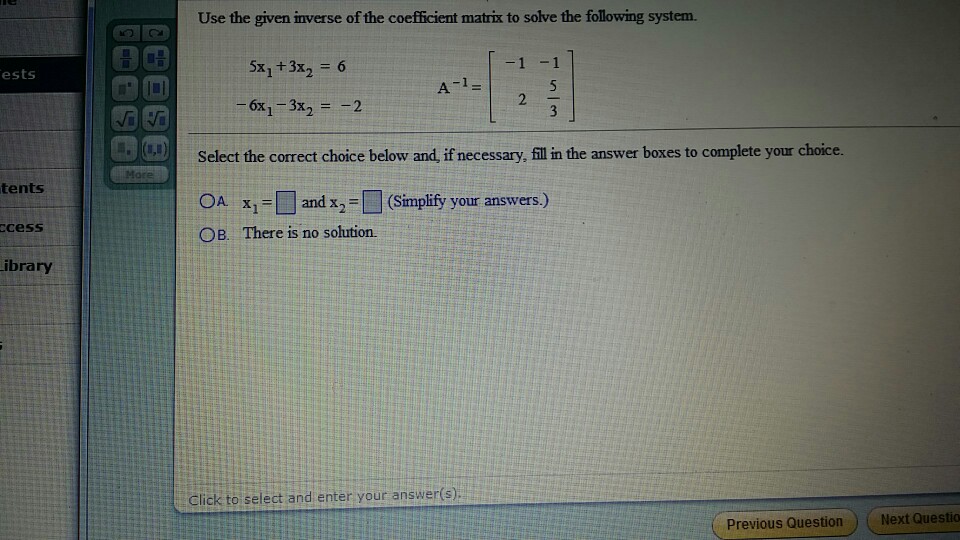
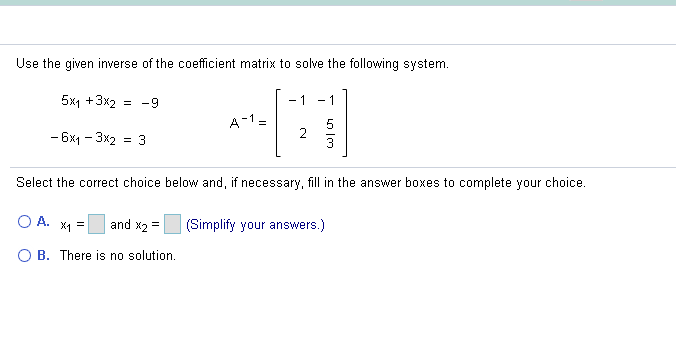
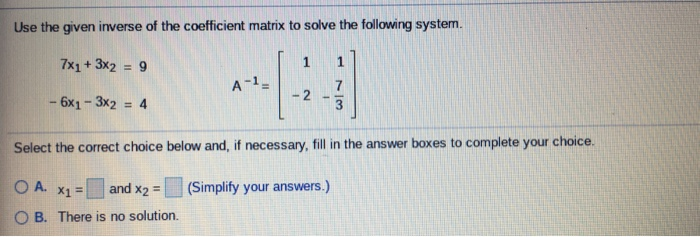
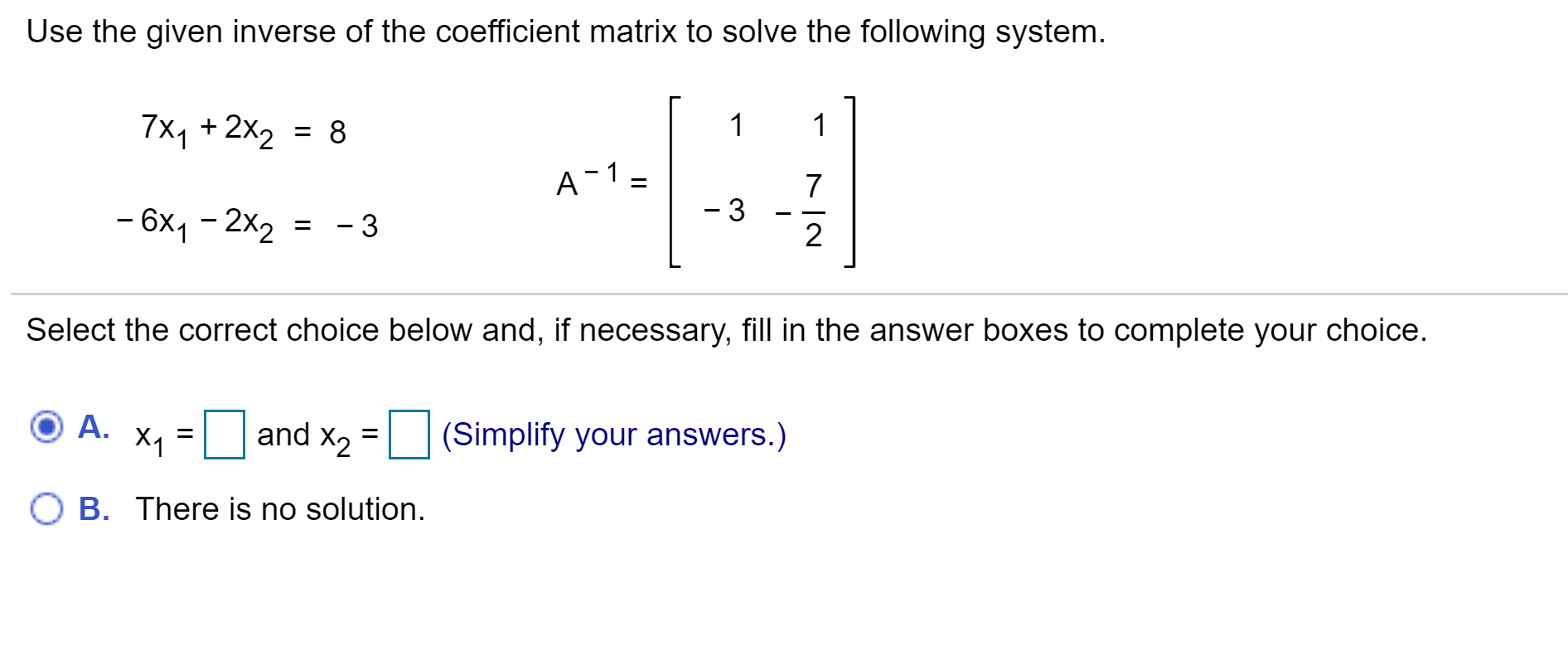
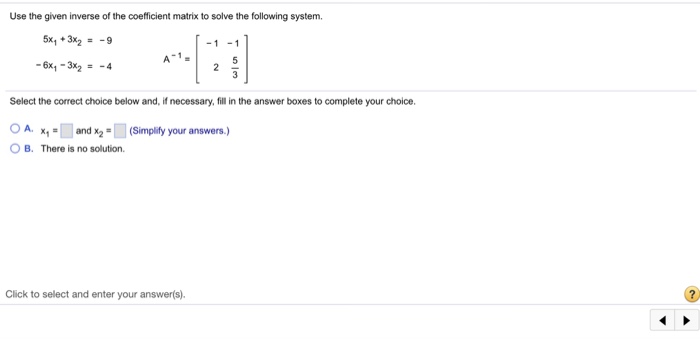
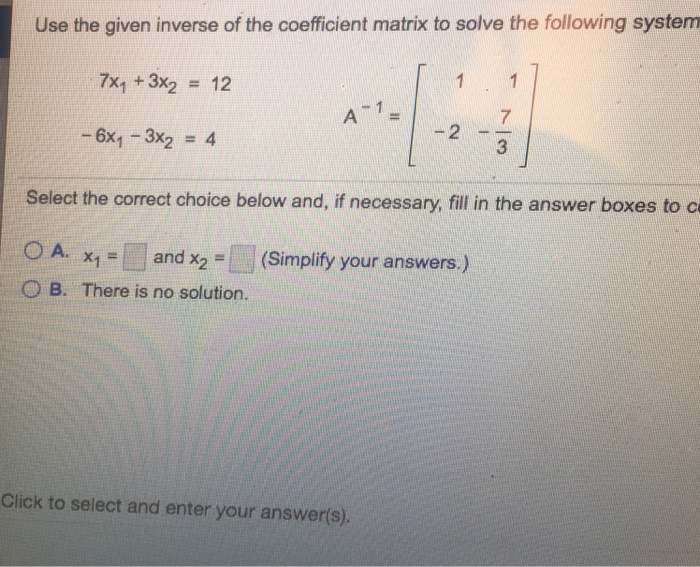
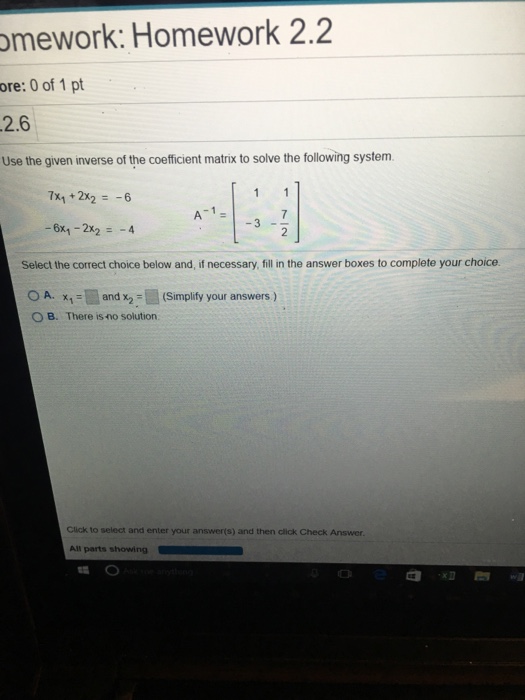
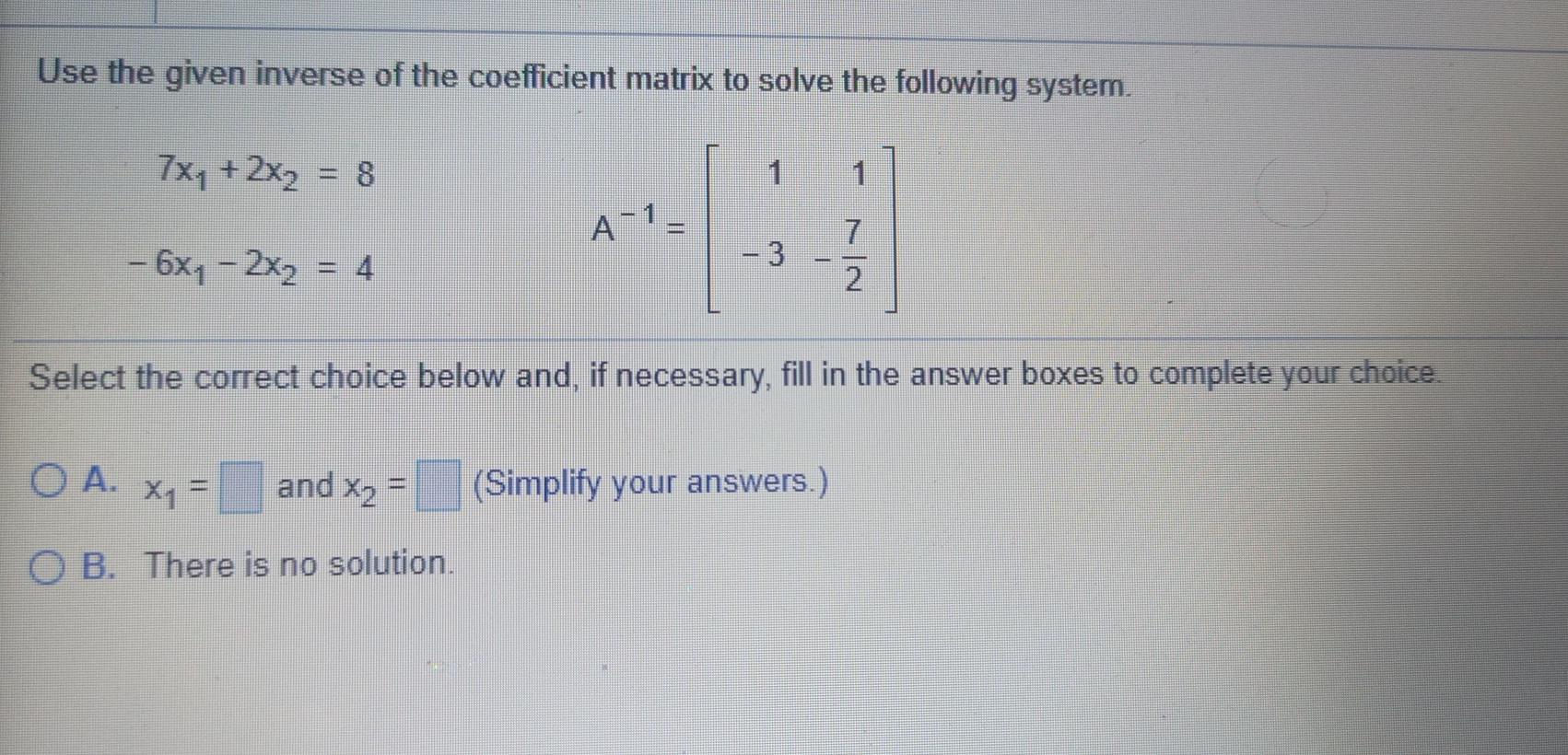
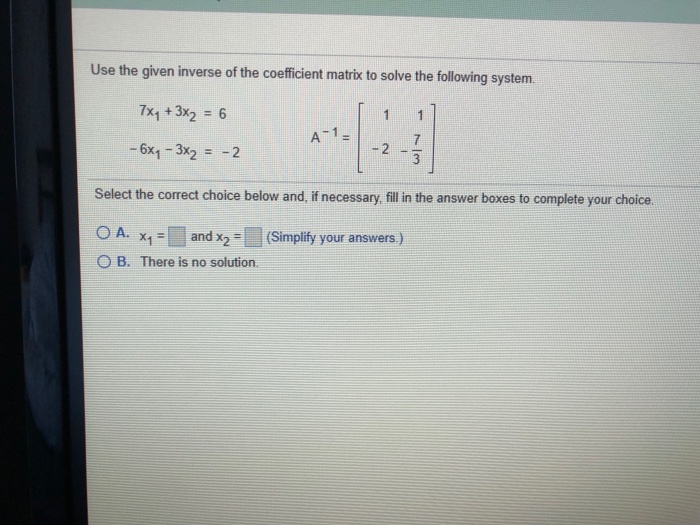
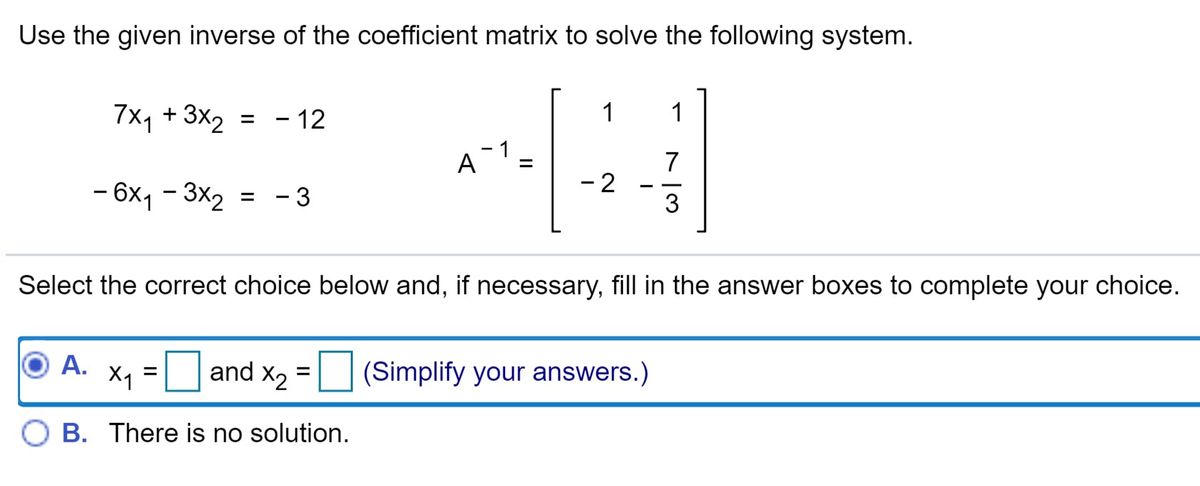
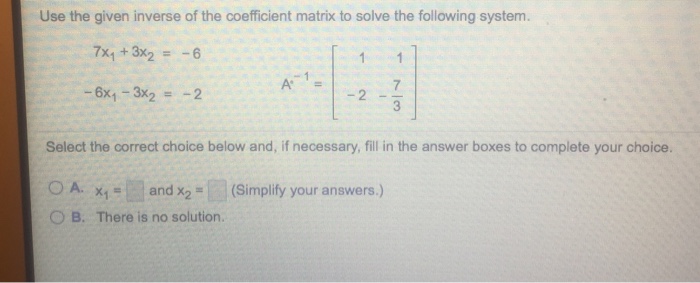
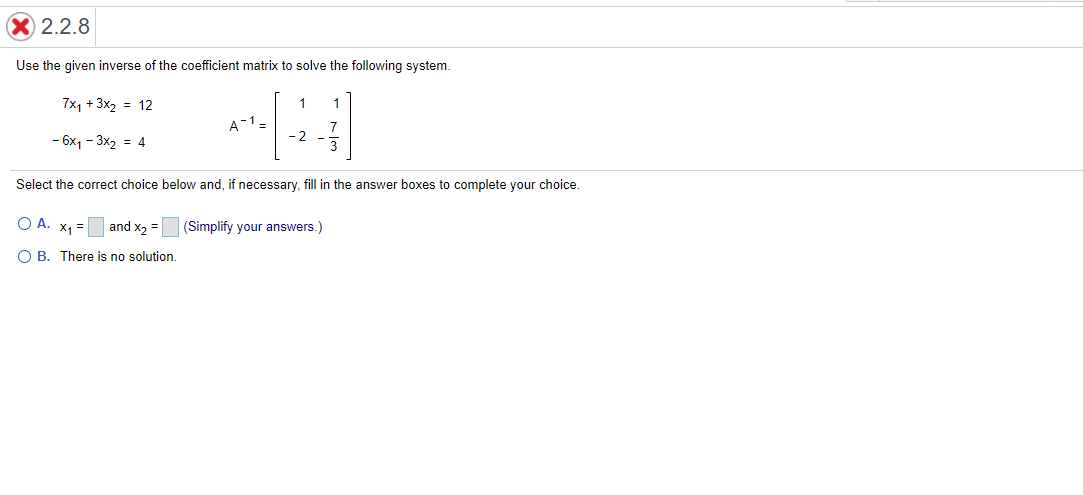
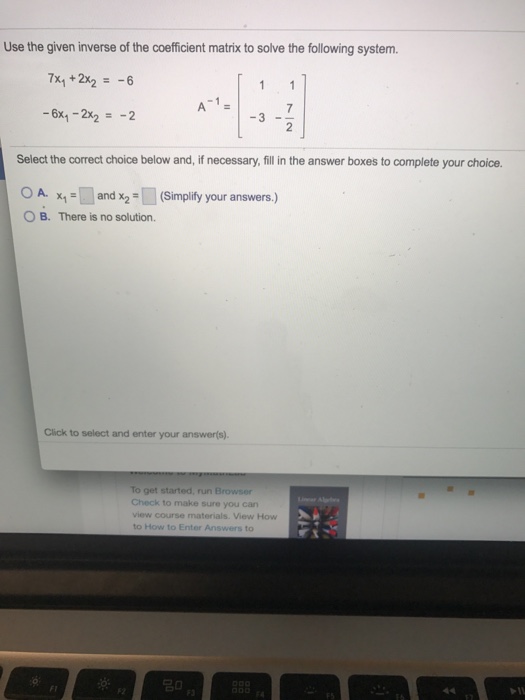
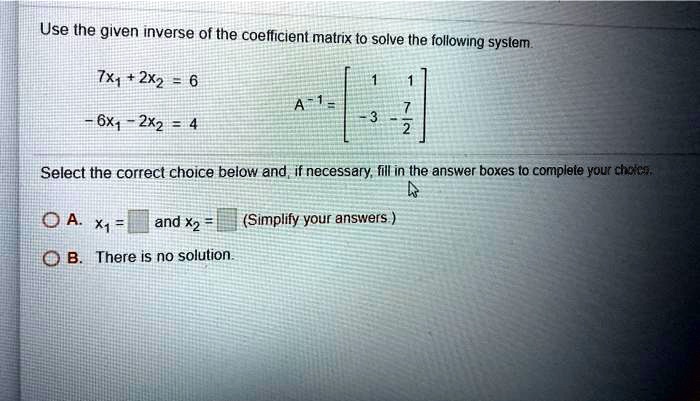
Use The Given Inverse Of The Coefficient Matrix To Solve The Following System
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
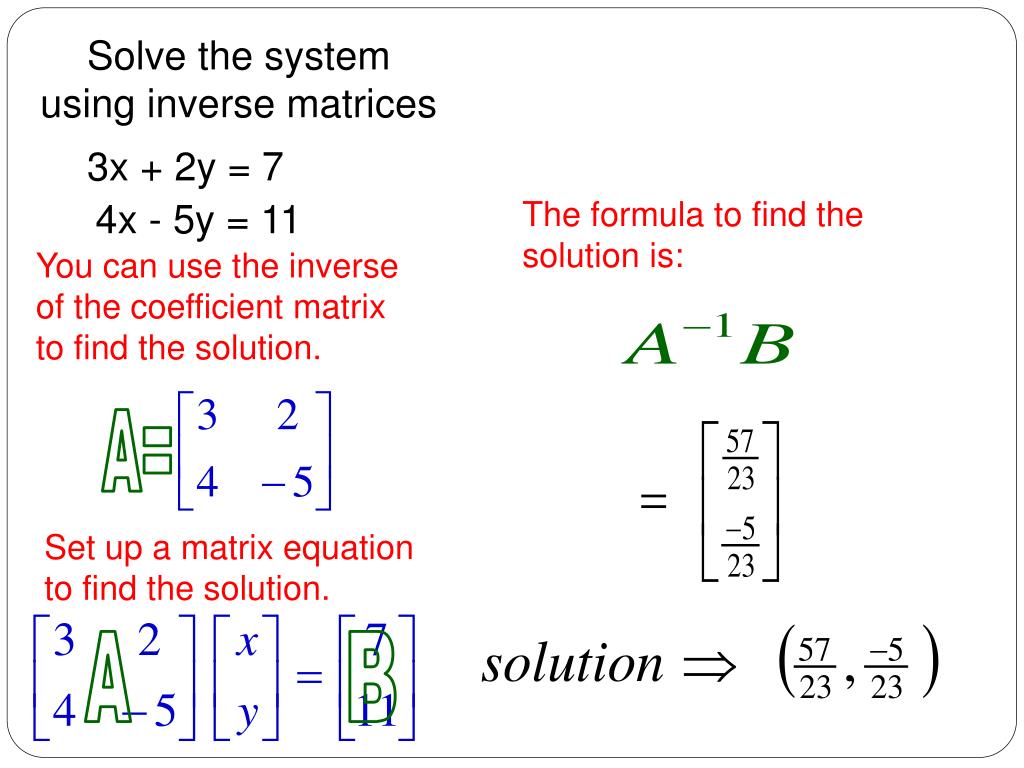
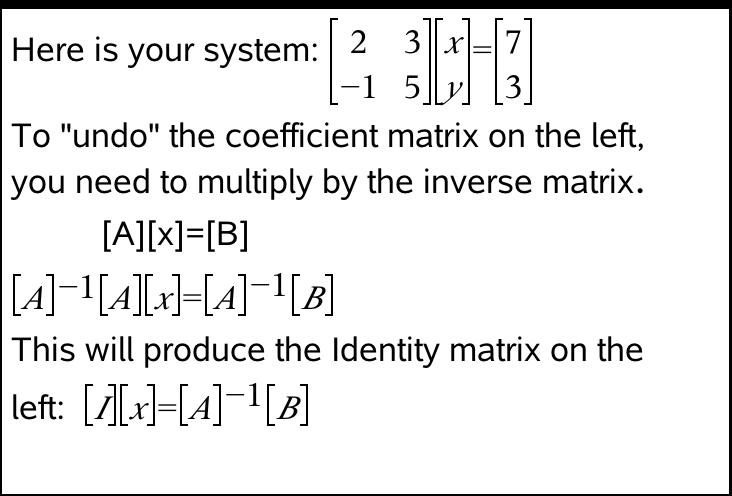
Use the given inverse of the coefficient matrix to solve the following system. By signing up youll get thousands of step-by-step solutions to your. The result vector is a solution of the matrix equation. To solve a system of linear equations using an inverse matrix let latexAlatex be the coefficient matrix let latexXlatex be the variable matrix and let latexBlatex be the constant matrix.
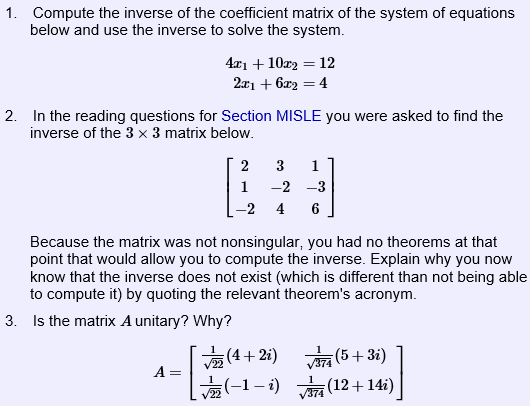
7 x 1 3 x 2 6. The coefficient matrix is beginbmatrix4-215endbmatrix To find the inverse use the formula A-11over ad-bcbeginbmatrixd-b-caendbmatrix Then multiply this inverse matrix by the vectors to find the answers. Then to the right will be inverse matrix.
Equivalent systems have the same solution. In this case a 4 b 3 c 10 and d 2. This result gives us a method for solving simultaneous equations.
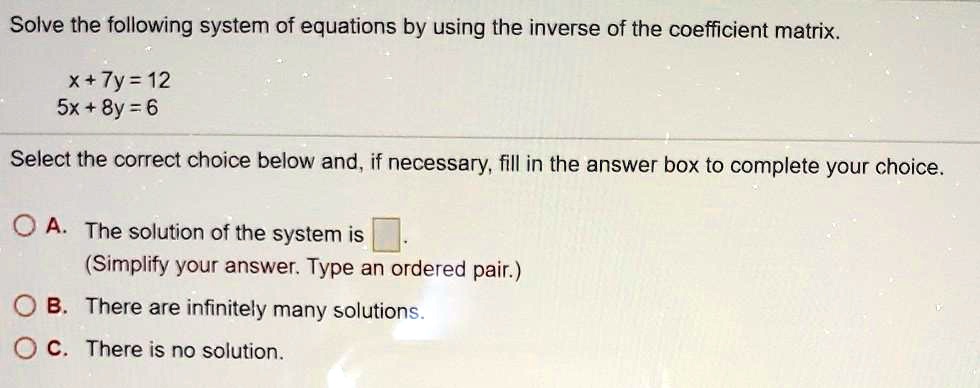
0A X1 and X2 Simplify your answers There is no solution. For instance you can solve the system that follows by using inverse matrices. X 1 x 2 x 3 x 4.
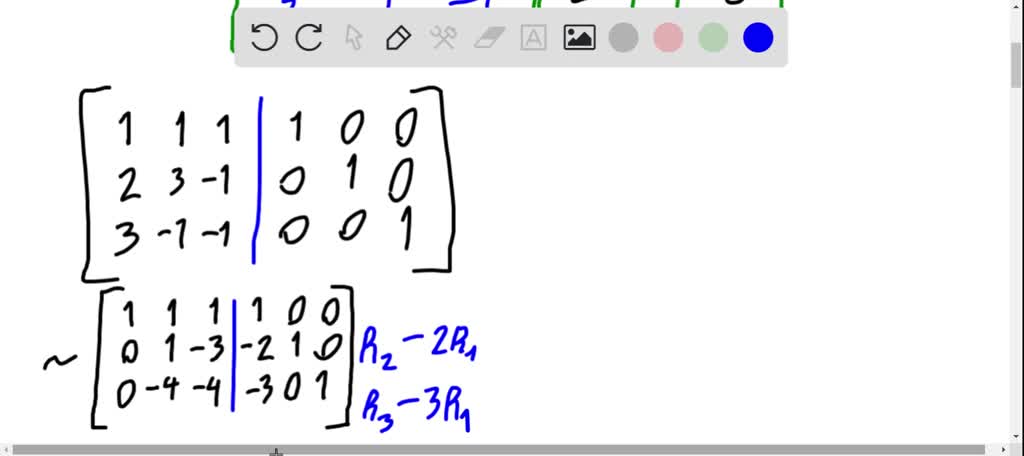
Multiply the inverse matrix by the solution vector. Algebra - Matrices-and-determiminant- SOLUTION. Hence the inverse matrix is.
Email me at this address if my answer is selected or commented on. Hence ad bc 22. Solution for Use the given inverse of the coefficient matrix to solve the following system.
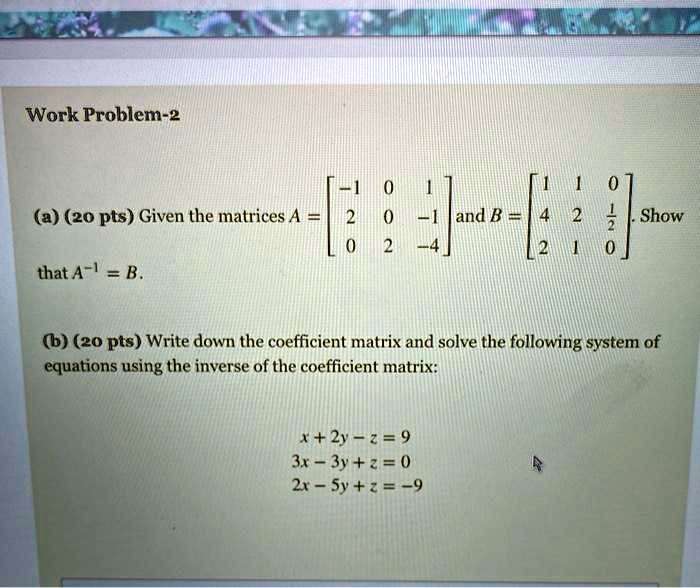
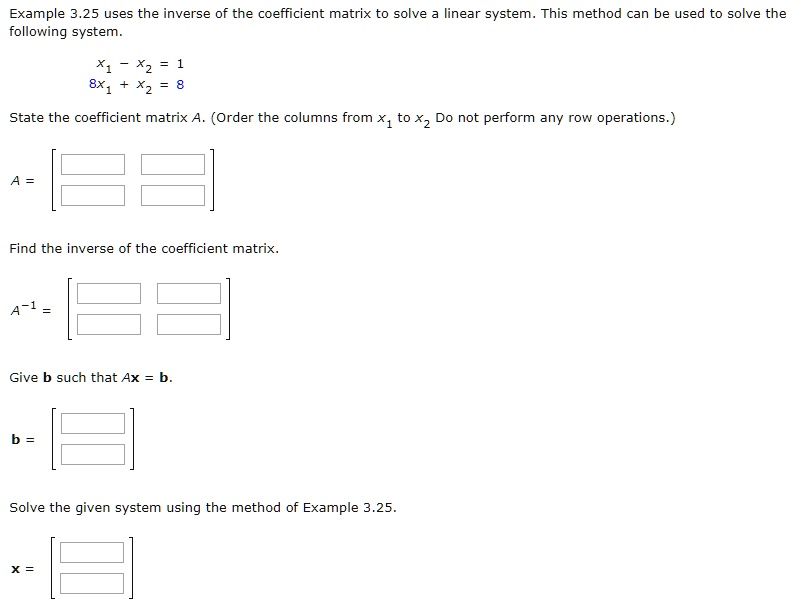
Furthermore IX X because multiplying any matrix by an identity matrix of the appropriate size leaves the matrix. A system of linear equations that has three equations and three variables is solved in this video using the inverse of the coefficient matrix and matrix mult.
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Solve The Following System 5x1 2x2 6 1 1 1 6x1 2x2 3 A S 3 Select The Corr Course Hero
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Use The Given Inverse Of The Coefficient Matrix To Solve The Following System 5x 1 3x 2 6 Plainmath
Answered Use The Given Inverse Of The Bartleby
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Answered Use The Given Inverse Of The Bartleby
Solved 2 2 8 Use The Given Inverse Of The Coefficient Matrix Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Solve He Following System 7x1 2x2 6x1 2x2 Select The Correct Choice Below And If Necessary Fill In The Answer Boxes 0 Complete
Solved Use The Given Inverse Of The Coefficient Matrix To Solve The Following System 5x1 2x2 4 1 A 1 3 2 Select The Correct Choice Below And If Necessary Fill In
Ppt Solving Systems Powerpoint Presentation Free Download Id 2732029
Solved Compute The Inverse Of The Coefficient Matrix Of The Chegg Com
Use An Inverse Matrix To Solve The Linear System Ppt Video Online Download
Solving A System Of Equations Using The Inverse Matrix Versus Using Gaussian Elimination Mathematics Stack Exchange
1
Solving A 3 X 3 System Of Equations Using The Inverse Youtube
4 Solve The Following System Of Linear Equations Using The Inverse Matrix Method 1 Y Homeworklib
Solving A System Of Equations Using The Inverse Matrix Versus Using Gaussian Elimination Mathematics Stack Exchange
Solving A System Of Linear Equations Using Inverses Youtube
Solve The Following System Of Equations By Using The I Itprospt
Solved Solve The Following Systems Of Equations By Using The Inverse Of The Coefficient Matrix Course Hero
Using Inverse Matrices Solving Systems You Can Use The Inverse Of The Coefficient Matrix To Find The Solution 3x 2y 7 4x 5y 11 Solve The System Ppt Download
Solved Work Problem 2 A 20 Pts Given The Matrices A And B Show That A 1 B 6 20 Pts Write Down The Coefficient Matrix And Solve The Following System Of Equations Using The
Solved A System Of Equations Is Given Together With The Chegg Com
Solved Use The Given Inverse Of The Coefficient Matrix To Solve The Following System 5x1 2x2 6 1 1 1 6x1 2x2 3 A S 3 Select The Corr Course Hero
Solve The Following System Of Equations By Using The I Itprospt
Solved 1 Use The Given Inverse Of The Coefficient Matrix To Chegg Com
Using The Inverse Of A Matrix To Solve A 3 By 3 System Of Linear Equations Youtube
Question Video Solving A Pair Of Simultaneous Equations Using Matrices Nagwa
Solved Example 3 25 Uses The Inverse Of The Coefficient Matrix To Solve Linear System This Method Can Be Used To Solve The Following System X1 Xz 9x1 X2 State The Coefficient Matrix
Using Matrix Inverse To Solve A System Of 2 Linear Equations Youtube
Answered Involving The Solving Of A System Of Bartleby
Solve The Following System Of Equations By Using The I Itprospt
Solve The System Of Linear Equations Using The Inverse Matrix Of The Coefficient Matrix Problems In Mathematics
Answered Involving The Solving Of A System Of Bartleby
3 8 Solving Systems Of Equations Using Inverse Matrices Youtube
Solving Linear Systems With Matrices Video Khan Academy
Solved Example 3 25 Uses The Inverse Of The Coefficient Matrix To Solve Linear System This Method Can Be Used To Solve The Following System X1 X2 8x X2 State The Coefficient
How Do I Use An Inverse Matrix To Solve A System Of Equations Socratic
1
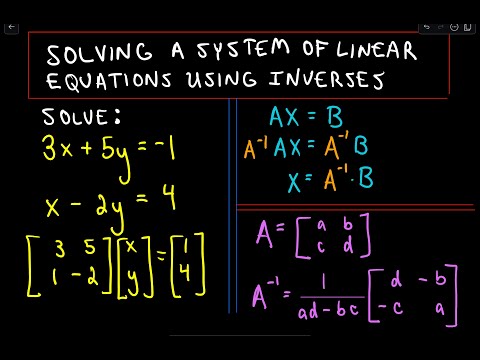
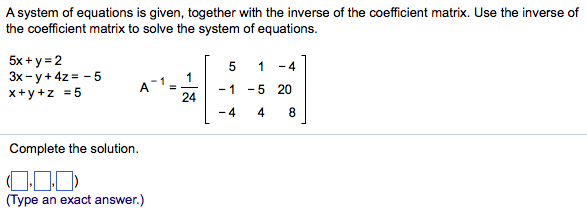
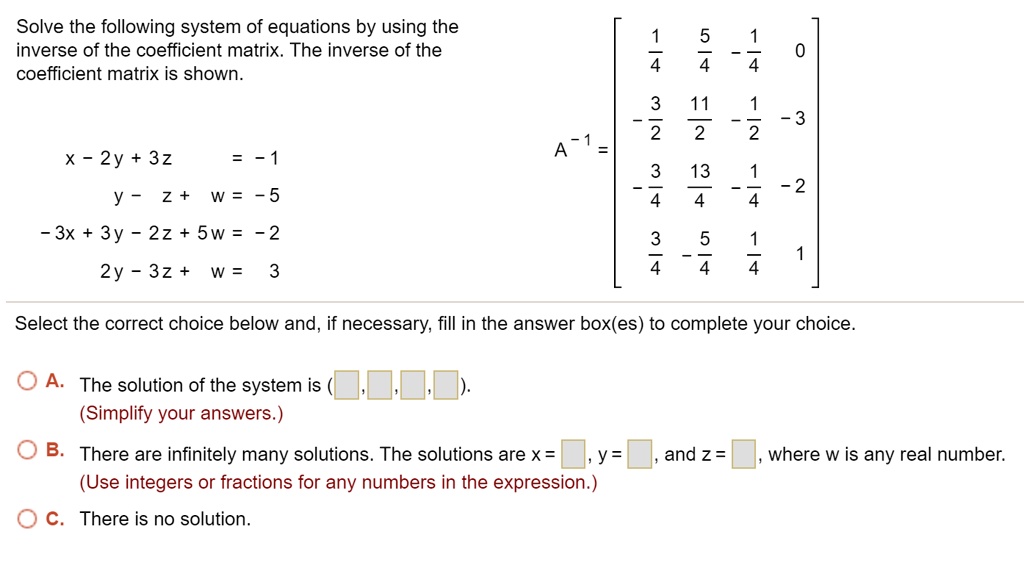
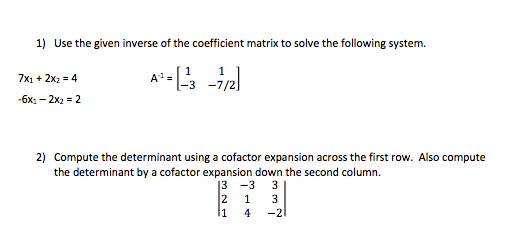
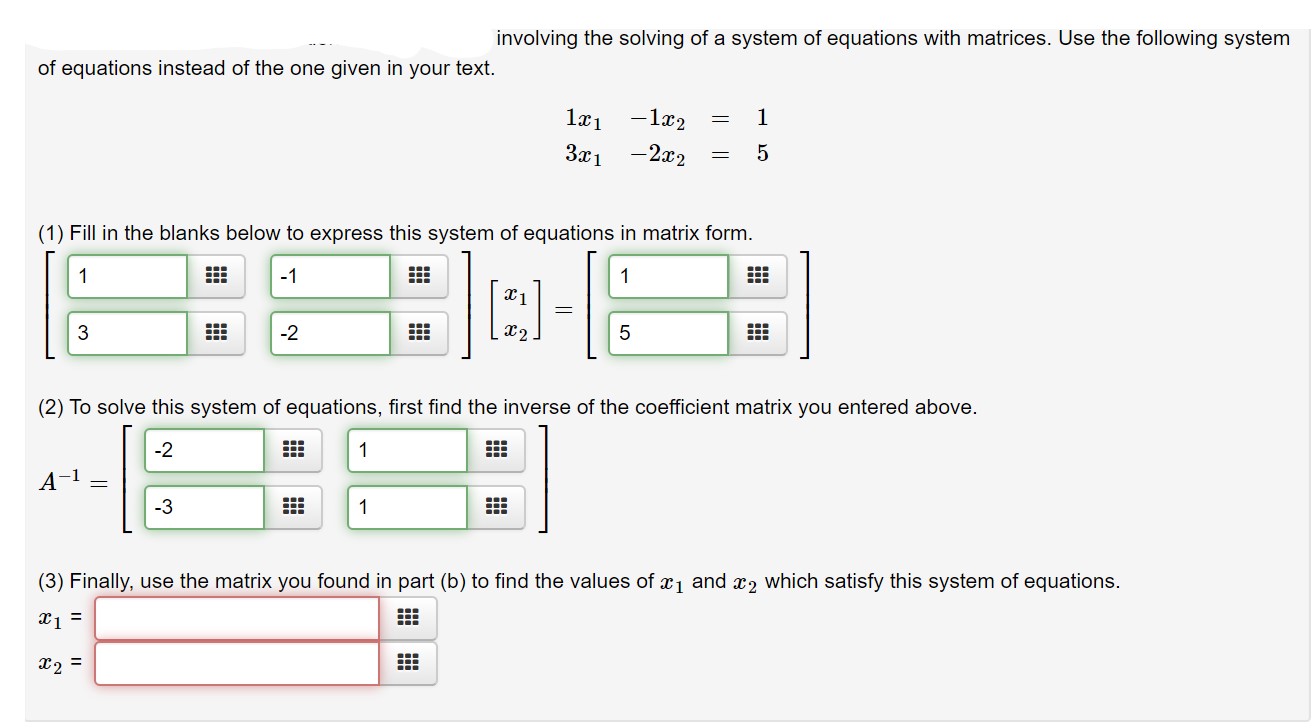
The inverse of the coefficient matrix may be used to solve a system of equations with two unknowns.
6 x 1 3 x 2 4. FInd the inverse of the coefficient matrix A. Solve Using an Inverse Matrix. Multiply the inverse matrix by the solution vector. Write the system in terms of a coefficient matrix a variable matrix and a constant matrix. Solve the system using the. You can use matrices and change your system in a matrix equation. The coefficient matrix is beginbmatrix4-215endbmatrix To find the inverse use the formula A-11over ad-bcbeginbmatrixd-b-caendbmatrix Then multiply this inverse matrix by the vectors to find the answers. X5yz11 3z12 2x4y2z8 All of the following operations yield a system which is equivalent to the original.
Solution for Use the given inverse of the coefficient matrix to solve the following system. The coefficient matrix is beginbmatrix4-215endbmatrix To find the inverse use the formula A-11over ad-bcbeginbmatrixd-b-caendbmatrix Then multiply this inverse matrix by the vectors to find the answers. You can use matrices and change your system in a matrix equation. Write the system in terms of a coefficient matrix a variable matrix and a constant matrix. First we need to find the inverse of the A matrix. And writing the coefficient matrix as A we have. Write the system as a matrix equation.
Post a Comment for "Use The Given Inverse Of The Coefficient Matrix To Solve The Following System"